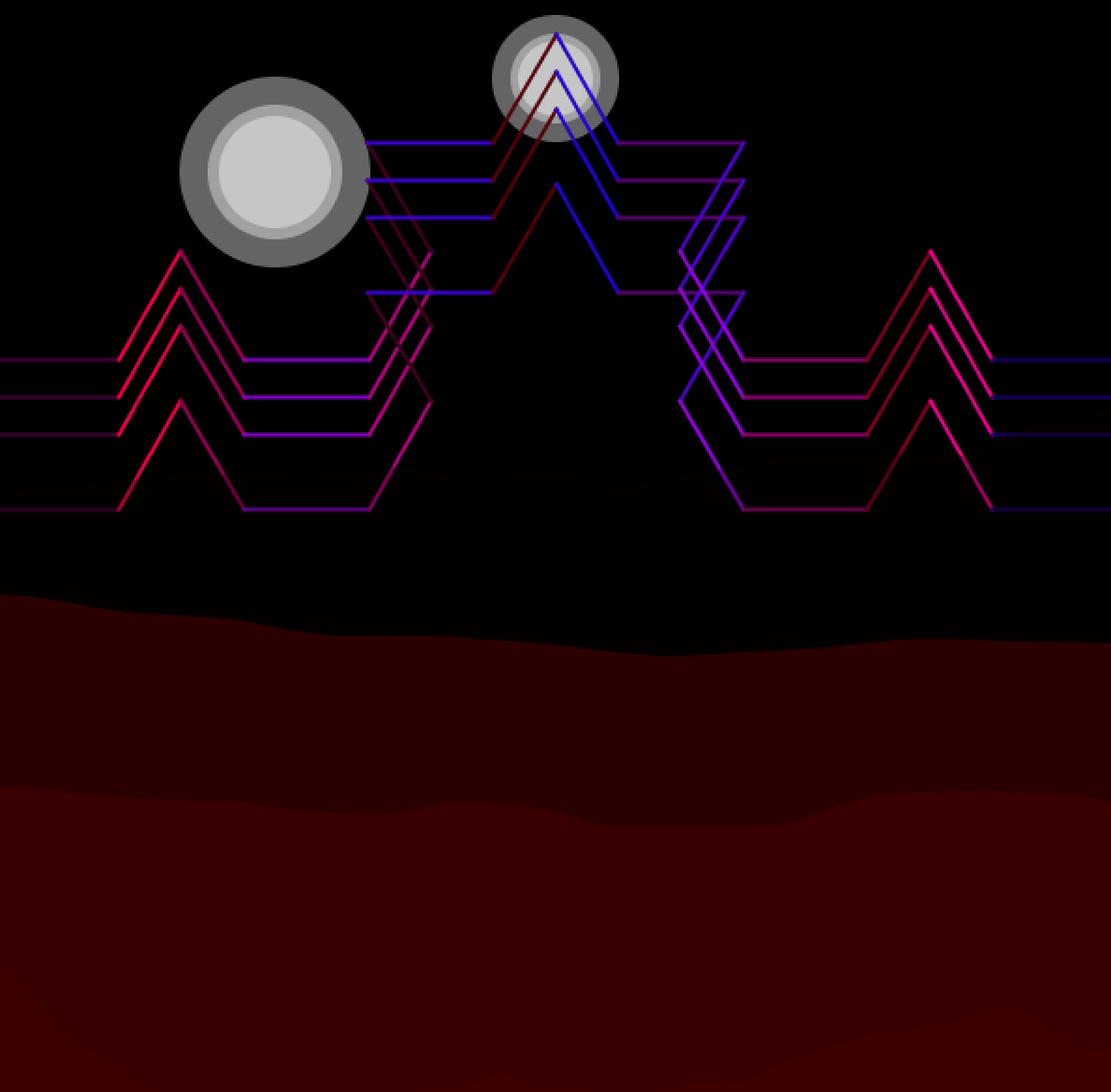
“Martian Moonscape”

Digital, recursive animation built with Processing

“…beautiful, damn hard, increasingly useful. That’s fractals.” - Benoit Mandelbrot

This piece was inspired by fractal geometry, found just as commonly in nature as they are in technology.

Fractals are recursive geometric figures that exhibit unfolding symmetry, resulting in patterns that look the same at every iteration, large or small. This aspect of self-similarity, as well as their prevalence in the natural world, is a source of fascination as well as mystery for both mathematicians and artists alike. How can something be continuous, but not differential, chaotic but organized, all at once?

Because of their inherently universal nature, the use of fractals in this sketch inspired me to move off of planet Earth and settle onto planet Mars. I wanted to depict a Martian moonscape, with the two Martian moons, Phobos and Deimos. To create further interest in the sketch, and to utilize fractal geometry, I wanted the viewer to suspend their disbelief and imagine a Martian “Aurora Borealis.”

I used the infamous Kotch curve to create my Aurora Borealis, with four horizontal lines recursively breaking down into smaller and smaller lines set at 60-degree angles to each other with randomized shades of red and blue. The result is a continuously shifting, regenerative pattern in the Martian night sky.

For the Martian landscape, I used a noise function to draw layered hills, or mountains, in various shades of red and at various amplitudes and decreasing opacity. This creates the illusion of distance, as if you are looking out over the alien, red planet at the vast night sky.